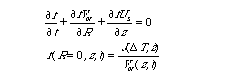
Structure of the upper boundary layer in sheet magma bodies is crucial factor in understanding pattern of magma convection and fractionation at relatively fast cooling rates. Worster and Huppert (1993) use ad hock assumption that in border two-phase zone crystals are immovable. It can be justified in metallurgical systems with column dendrite fronts originated at directional solidification but not in magmatic ones. From general point of view below capturing front suspension zone with settling crystals is anticipated by Marsh (1989).
To describe crystallization we use approximation of two-component system with two phases of constant composition of eutectic type. Then in the volume crystallization of one above eutectic phase (A) takes place and both phases are formed at Stefan front at eutectic temperature. Model includes equations of conservation for both components in the melt and for phase A, Stokes equation for relative crystal velocity and heat transport equation. Case of constant settling rate was considered before (Simakin et al., 1994). Solution is proven to describe temperature and composition distribution in more complex situations. For the variable due to growth crystal sizes evolutionary equation for Crystal Size Distribution (CSD) is used instead of crystal conservation one:
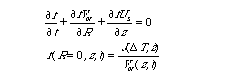
where J(DT,z) is nucleation rate. For preliminary analysis of our model we study steady-state with fixed heat extraction rate Q at eutectic front. Heterogeneous nucleation at preexisting solid particles in the outer part of boundary layer is assumed. Settling velocity Us is represented as product of Stokes velocity (S=aR2) factor accounting melt counterflow and viscosity increase due to crystal present Us=S(1-e)m(e). At favourable values of kinetic parameters undercoolings developed in boundary layer are small so total volume crystallization rate (G) can be predicted using our equilibrium solution (Simakin et al., 1994). In this mixed approach (analytical solution for G and numerical solution of equation for CSD in moving coordinates at fixed volume solidification rates) we find condition of escaping for crystals of
phase A. Escaping occurs if nondimensional parameter g=a×Ub-1n-2/3 exceeds critical level about 20 (where Ub solidification front velocity proportional to Q, a- proportionality coefficient in Stokes relation, n - volume content of solid seeds in the magma). Generally our results are in agreement with simpler estimates of Mangan and Marsh (1992).
Work was partially supported by DFG during visit to Frankfurt Institut for Meteorology and Geophysics and Russian grant RFBR #93-05-8190.
Mangan, M. & Marsh, B., J. Geology 100, 605-620 (1992).
Marsh, B., Annu. Rev. Earth Planet. Sci. 17, 439-474 (1989).
Simakin, A., Schmeling, H. & Trubitsyn, V., Earth Planet. Sci. Lett. 126, 333-349 (1994).
Worster, G. & Huppert, H., J. Geophys. Res. 98, 14,075-14,090 (1993).