Convective motions in general are characterized by an interaction between flow and transport processes. While transport is determined by the velocity field in any dynamic case, the effect of transport on fluid flow is an exceptional case. Then the non-constant distribution of heat or salt may influence fluid density and/or viscosity. Temperature differences are the most studied origin of convection, when a fluid is heated from below. Salinity gradients on the other hand can induce convection as well, when saline fluid are located above fresh water.
The basic laws governing the motions below the surface are: fluid mass conservation, energy conservation and Fourier's Law for the thermal case, salt mass conservation and (generalized) Fick's Law in the saline case. Density changes are given by a state-equation, while Darcy's Law describes fluid flow in porous media. A combination of these basic principles leads to coupled partial differential
equations.
Free convective flow under idealized conditions is characterized by two dimensionless numbers. (1) The Rayleigh-no. Ra is a combination of physical parameters.
(2) The Nusselt-no. Nu is proportional to the mean heat transfer in vertical direction. While Ra provides a characterization of the system from it's physical properties, Nu characterises it's behaviour. Usually the physical parameters can be obtained directly or indirectly from measurements and it is questioned, what the mean transfer will be under the given conditions. The inverse problem has the response of the system and aims to determine one of the parameters. In both cases it is necessary to understand the connection between Nu- and Ra-numbers.
The classical result is, that convective motions need a Rayleigh-no. above a marginal value, which in the porous media case is 4p2. In subcritical cases holds: Nu=1; whereas in supercritical cases the heat transfer is increased: Nu>1. The relationship between Nu and Ra depends on the convective flow pattern.
A series of numerical simulations of the transient development of convection have been carried out. The computer calculations are based on a finite-difference discretisation (CIS central in space, CIT central in time) of the differential equations. Details of the computer code FAST_C(2D) have been described in (Holzbecher, 1991). For each simulation run the Rayleigh-number was fixed. Depending on initial conditions different steady state patterns have been approached. The branches of the steady solution (with different cell aspect ratio k) have been traced in the following way: the steady state solution for determined values of k and Ra was used as an initial value for a simulation run with a slightly changed value for Ra. The new steady state has again been taken as initial state for another Ra. In that way it was possible to follow the different branches up to Ra=500 and down to Nu<2.
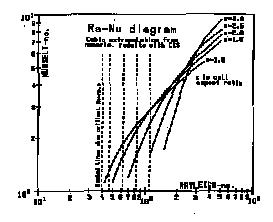
The resulting figure, given below, shows the different branches of steady state solutions in the phase space - in the vicinity and far from the marginal state. Five branches of one-layered (2D-) eddy solutions for different cell aspect ratios k were examined. The height to length ratios k for the steady state cells are 1., 1.5, 2., 2.5 and 3. All curves are of parabolic shape. The footpoint of the square cell branch (k=1.) is at (Ra=Racrit, Nu=1), where Racrit is the critical Rayleigh-No. The footpoints of the other branches are identical to the corresponding critical values Racritk which can be derived analytically (Holzbecher, 1991). For higher values of Ra the square cell branch becomes the one with lowest Nu-number. With increasing Ra branches with higher cell aspect ratio will have higher heat transfer.
Platzman (1965) stated that the solution with maximum heat transfer is preferred. Thus with increasing Rayleigh-numbers the convective patterns show slimmer cells, which in fact has been observed in laboratory experiments.
Holzbecher, E., Modellierung von Dichteströmungen, TU Berlin, IWAWI Mitlg 117 (1991).
Platzman, G.W., J. Fluid Mech. 23 (1965).
Fig. 1: Ra-Nu-diagram for 5 convection patterns with different cell aspect ratio *.